Least Common Ancestor

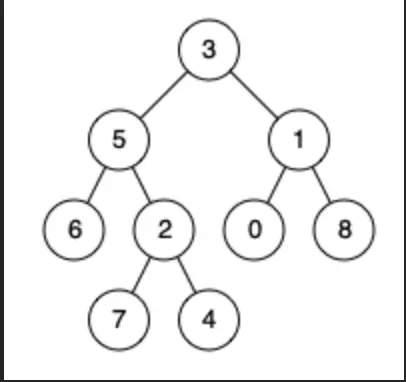
Given a binary tree, find the lowest common ancestor (LCA) of two given nodes in the tree.

According to the definition of LCA on Wikipedia: “The lowest common ancestor is defined between two nodes p and q as the lowest node in T that has both p and q as descendants (where we allow a node to be a descendant of itself).”

Example 1:

Input: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 1 Output: 3 Explanation: The LCA of nodes 5 and 1 is 3.

Example 2: Input: root = [3,5,1,6,2,0,8,null,null,7,4], p = 5, q = 4 Output: 5 Explanation: The LCA of nodes 5 and 4 is 5, since a node can be a descendant of itself according to the LCA definition.

Example 3:

Input: root = [1,2], p = 1, q = 2 Output: 1

Constraints:

• The number of nodes in the tree is in the range [2, 10^5].
• -10^9 <= Node.val <= 10^9
• All Node.val are unique.
• p != q
• p and q will exist in the tree.

Solution:

class Solution {
    public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
        if(root == null){
            return root;
        }
		//If the current node is either `p` or `q`, return the current node (`root`) because 
		//one of the nodes to be found is encountered,
		//**** baaki doosra node toh kisi na kisi sub-tree mei hoga hi.. Jo pehle mila woh King.
        if(root.val == p.val || root.val == q.val){
            return root;
        }
 
        TreeNode lA = lowestCommonAncestor(root.left, p, q);
        TreeNode rA = lowestCommonAncestor(root.right, p, q);
 
		//If both left and right recursive calls return non-null values, 
		//it means `p` and `q` are found in different subtrees of the current 
		//node (`root`). Hence, the current node is their lowest common ancestor.
        if(lA != null && rA != null) return root;
 
		//If only one of the left or right recursive calls returns a non-null value, 
		//it means both `p` and `q` are located in that subtree. 
		//Therefore, return the non-null value.
        return lA == null ? rA : lA;
    }
}